Section: New Results

3D Data Rendering and Visualization

Soft shadows

Participant : Gaël Guennebaud.

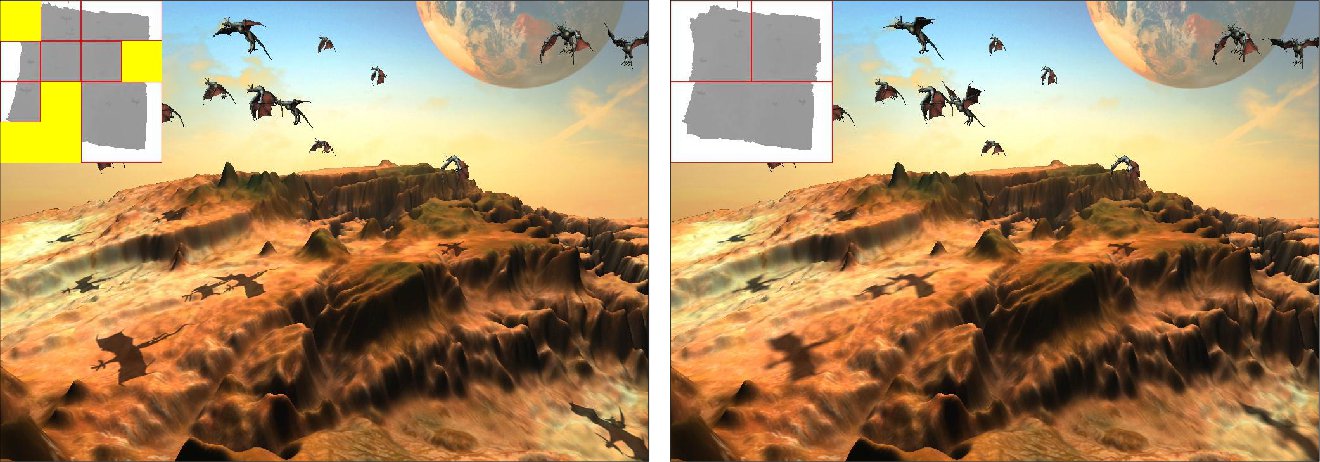
Shadows are a fundamental visual effect which both increase the level of realism of a 3D scene, and help to identify spatial relationships between objects. This latter observation makes them particularly important in the context of interactive 3D applications. Generating high quality soft shadows in real-time is still an open challenge. In the continuity of our previous collaboration with the State Key Lab of CAD&CG of Zhejiang University (China)  [39] , we developed a perceptually based metric dedicated to the prediction of ideal shadow map resolutions [16] . This metric allows us to adaptively generate shadow map tiles. As a result, we managed to render wide and complex exterior scenes with high quality while maintaining high performance (see figure 2 ).

Figure 2. Our soft shadow rendering system generates adaptive shadow map tiles and can therefore render softer shadows (right @ 25 fps) faster than their hard shadow counter part (left @ 15 fps).

Synthesis and control of breaking waves

Participants : Nicolas Maréchal, Pascal Barla, Gaël Guennebaud, Patrick Reuter.

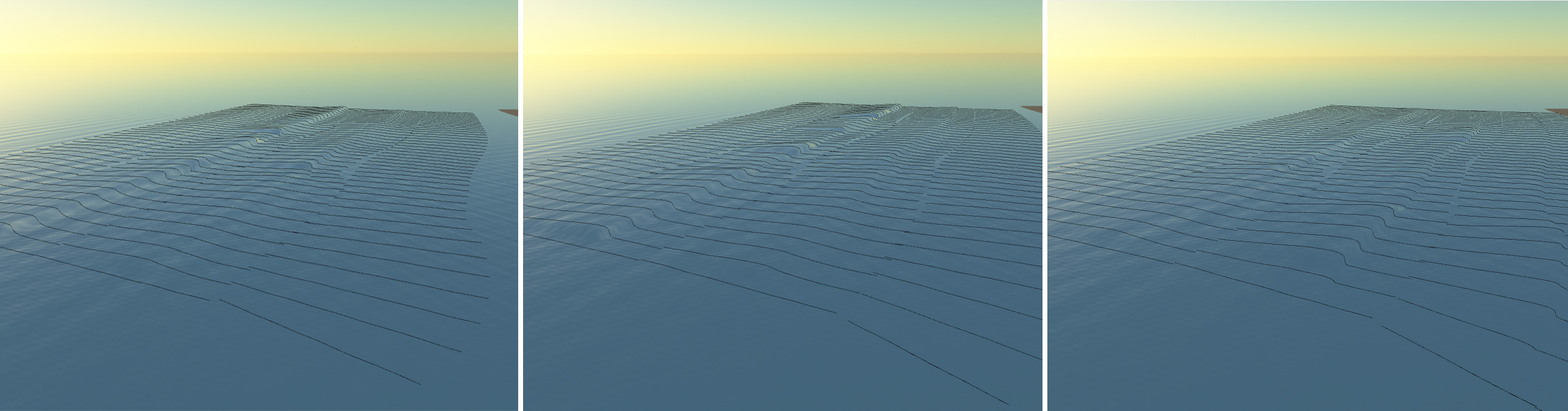
Modeling complex breaking waves over arbitrary bathymetry is a tedious problem. Currently, most of the existing methods are based on physical simulations by solving the navier-stokes equation. Controlling the shape of breaking waves is almost impossible with such approaches, and the simulation does not run in real-time. In order to overcome these limitations, we propose a phenomenological approach based on a real-time simulation using airy's wave theory. Our system handles phenomena such as shoaling, refraction and grouping (see figure 3 ), and the rendering style can be adapted by the user.

Figure 3. Breaking waves generated using our system over an arbitrary bathymetry. 2D wave shape profiles are shown for better legibility.

Analysis and visualization of surface relief

Participants : Lucas Ammann, Pascal Barla, Gaël Guennebaud, Xavier Granier, Patrick Reuter.

Given a base surface with relief, we developed an analysis technique that leverages the complexity found in detailed 3D models for illustrative shading purposes. The key originality of our approach is to extract the relief features such as concavities, convexities and inflections at multiple scales and directions using local cubic-polynomial fitting. We use this information to guide a variety of shading techniques. Our approach is parametrization-free and meshless, allowing for a wide variety of applications ranging from scientific visualization to special effects for the movie industry.